What If The Discriminant Is Negative | What Will Happen If A Discriminant Is Negative?

If the discriminant of a quadratic equation is negative, the roots of the equation are complex.

Let’s break down why this happens. The discriminant is a part of the quadratic formula, which helps us find the solutions (or roots) of a quadratic equation. The quadratic formula is:

x = (-b ± √(b² – 4ac)) / 2a

Where:

a, b, and c are the coefficients of the quadratic equation (ax² + bx + c = 0).

The discriminant is the part under the square root: b² – 4ac.

Here’s the key:

* When the discriminant is positive, the square root results in a real number. This means we get two distinct real roots.
* When the discriminant is zero, the square root becomes zero. This leads to a single real root.
* When the discriminant is negative, we have the square root of a negative number. This results in an imaginary number (specifically, a complex number).

Complex numbers are numbers that involve the imaginary unit i, where i² = -1. Complex roots come in pairs, with one being the conjugate of the other. A conjugate is formed by simply changing the sign of the imaginary part.

For example: If one root is 2 + 3i, the other root would be 2 – 3i.

So, when the discriminant is negative, it signals that the quadratic equation has two complex roots instead of real roots. These roots are not directly visible on a standard number line but exist in the complex plane.

Remember, even though these roots are not real numbers, they still represent valid solutions to the quadratic equation. They are just expressed using imaginary numbers.

Let’s talk about what happens when the discriminant of a quadratic equation is negative.

You’re probably familiar with the quadratic formula, right? It’s used to find the solutions (also called roots) of a quadratic equation in the form ax² + bx + c = 0. The discriminant, which is the part under the square root sign (b² – 4ac), tells us a lot about the nature of the roots.

If the discriminant is negative, the roots are imaginary.

Imaginary roots might sound a bit mysterious, but they’re just numbers that involve the imaginary unit, i, which is defined as the square root of -1. Imaginary roots are often expressed in the form a + bi, where a and b are real numbers.

Let’s break this down a little more. Imagine you’re trying to find the square root of a negative number. Since the square of any real number is always positive, you can’t find a real number that, when squared, gives you a negative result. That’s where imaginary numbers come in! They extend the number system beyond real numbers to allow us to work with square roots of negative numbers.

Think of it like this: The real number line represents all the numbers you’ve worked with before (positive, negative, and zero). Imaginary numbers add another dimension to this line, creating a complex plane. This plane allows us to represent both real and imaginary numbers.

So, when the discriminant is negative, the quadratic equation has no real solutions. Instead, it has two imaginary solutions (which are always complex conjugates of each other, meaning they have the same real part but opposite imaginary parts). This means there are no points on the x-axis where the graph of the quadratic equation intersects.

Let’s talk about the discriminant and how it helps us understand the roots of a quadratic equation.

You’re right, if the discriminant is positive, you have two real roots. And, if it’s zero, you have one real root. But what happens when the discriminant is negative?

Well, in this case, you have no real roots. This means the equation doesn’t have any solutions that are real numbers.

Think of it this way: the discriminant tells you how many times the parabola representing the quadratic equation crosses the x-axis. When the discriminant is positive, the parabola crosses the x-axis in two places. When it’s zero, the parabola touches the x-axis at one point (like a tangent line). But when the discriminant is negative, the parabola doesn’t intersect the x-axis at all.

This is because the discriminant is the part of the quadratic formula that’s under the square root sign. And, you can’t take the square root of a negative number and get a real number. The solutions, in this case, would be complex numbers (numbers with an imaginary component).

Let’s look at an example. Imagine you have the equation: x² + 2x + 5 = 0. The discriminant of this equation is 2² – 4 * 1 * 5 = -16. This negative value means the equation has no real roots.

Understanding the discriminant can be very helpful in solving quadratic equations. It gives you a quick way to determine the nature of the roots (real or complex) without having to solve the entire equation.

Let’s talk about what happens when the discriminant is zero. When the discriminant is zero, you have exactly one real root. This means that the quadratic equation has a single solution. Think of it like a parabola touching the x-axis just once at its vertex.

But remember, a quadratic equation usually has two solutions. So, what’s going on here? The single root is actually a double root, which means it appears twice in the solution set. You can think of it as two roots that happen to be the same value.

Let’s break it down with an example:

Imagine the equation x^2 – 4x + 4 = 0. You can use the quadratic formula to find the roots:

x = (-b ± √(b^2 – 4ac)) / 2a

Plugging in the values (a=1, b=-4, c=4), we get:

x = (4 ± √((-4)^2 – 4 * 1 * 4)) / 2 * 1

Simplifying:

x = (4 ± √(0)) / 2

x = 2

As you can see, the discriminant (b^2 – 4ac) is zero, and we end up with just one solution: x = 2. This solution is a double root, meaning it’s repeated twice.

This is a key concept for understanding quadratic equations. When the discriminant is zero, we get a double root, and the parabola representing the equation just touches the x-axis at that single point.

Don’t worry if you get a negative discriminant! It just means you’ll have two complex solutions instead of real ones. Let me break that down for you.

When we solve quadratic equations, we often use the quadratic formula. This formula has a part called the discriminant – that’s the part under the square root sign. The discriminant tells us a lot about the nature of the solutions to the equation.

If the discriminant is positive, we get two real solutions, meaning they’re regular numbers like 3, -5, or 1.75. If the discriminant is zero, we get one real solution (which is actually two identical solutions). But if the discriminant is negative, we’re in the realm of complex numbers.

Complex numbers involve the imaginary unit, represented by the letter i, where i² = -1. The complex solutions will look like this: a + bi, where a and b are real numbers. This means the solutions involve both a real part (a) and an imaginary part (b).

So, while the equation might not have real solutions, it still has solutions. They just exist in the world of complex numbers!

Let’s talk about what happens when D (the discriminant) is negative in a quadratic equation. When D is negative, the quadratic equation doesn’t have any real roots. This means the graph of the equation won’t intersect the x-axis.

Think about it this way: The roots of a quadratic equation are the points where the graph crosses the x-axis. If the graph doesn’t cross the x-axis, it means there are no real numbers that satisfy the equation.

Here’s the thing: when D is negative, the quadratic formula gives you imaginary numbers as the solutions. Remember that imaginary numbers are numbers that include the imaginary unit i, where i is the square root of -1.

For instance, if the quadratic formula gives you a solution like 3 + 2i, it means the roots of the equation are imaginary. The graph of the equation will be a parabola that doesn’t intersect the x-axis at all.

Think about it this way: the quadratic formula helps you find the values of x that make the equation equal to zero. When D is negative, the formula leads you to square roots of negative numbers. Since we can’t take the square root of a negative number in the real number system, you end up with imaginary numbers instead of real roots.

So, even though the quadratic equation has no real roots when D is negative, it does have two complex roots, which are expressed using imaginary numbers. This is a key concept to grasp when working with quadratic equations.

Let’s dive into what a negative discriminant means in the world of quadratic equations!

If you’re working with the quadratic formula, you know the discriminant is the part under the radical: b² – 4ac. When this value turns out to be negative, it signifies a really interesting thing: there are no real solutions to the quadratic equation.

Think of it this way: you can’t take the square root of a negative number and get a regular, everyday number. The solutions to the quadratic equation in this case are called complex numbers. They involve the imaginary unit i, where i² = -1. Complex numbers are super cool, but they’re not something you can easily plot on a regular number line graph.

Let’s break down why this happens. When you’re solving a quadratic equation, you’re essentially looking for the points where the graph of the equation crosses the x-axis. These points are the roots or solutions of the equation. If the discriminant is negative, it means the parabola (the graph of a quadratic equation) never touches the x-axis.

Think of it like this: If you throw a ball straight up in the air, it will eventually come back down. The point where the ball reaches its highest point before coming down is like the vertex of the parabola. If the ball never touches the ground, it means it never crossed the x-axis. Similarly, a negative discriminant means the graph of the quadratic equation never touches the x-axis.

So, while a negative discriminant might seem like a roadblock, it’s actually a sign that you’re dealing with something a little more complex and fascinating!

Let’s talk about what happens when the discriminant is a positive number!

If the discriminant is positive, it means your quadratic equation has two distinct real solutions. Think of it like this: the discriminant acts like a guide, telling you how many times the parabola representing your equation crosses the x-axis. When the discriminant is positive, it means that parabola crosses the x-axis in two different places, leading to two unique solutions.

Let me break it down further. The discriminant is a part of the quadratic formula, which is a tool to find the solutions (or roots) of a quadratic equation. The quadratic formula looks like this:

x = (-b ± √(b² – 4ac)) / 2a

The part under the square root sign, b² – 4ac, is the discriminant. Now, when the discriminant is positive, the square root of a positive number gives you another positive number. This means you’ll have two possibilities when you use the quadratic formula: one with a plus sign before the square root and another with a minus sign. These two possibilities lead to two different solutions for your equation.

Think of it as finding the places where your parabola touches the ground. When the discriminant is positive, you’ll have two points where your parabola crosses the x-axis. These points are your solutions, the places where your equation is equal to zero.

Let’s talk about what a positive discriminant means! When you’re working with quadratic equations, the discriminant is a super helpful tool to understand the nature of the solutions.

A positive discriminant tells us that the quadratic equation has two distinct real number solutions. This means that the parabola representing the quadratic will intersect the x-axis at two different points.

Think of it this way: imagine you’re throwing a ball in the air. The path of the ball is a parabola. If the ball hits the ground twice, that’s like the quadratic having two real solutions.

What about a discriminant of zero? That indicates the quadratic has a repeated real number solution. The parabola touches the x-axis at just one point.

And what about a negative discriminant? This means neither of the solutions are real numbers. In this case, the parabola doesn’t intersect the x-axis at all.

Let’s dive a bit deeper into what a positive discriminant means.

Remember that a quadratic equation is typically written as:

ax² + bx + c = 0

The discriminant is calculated using the coefficients of the quadratic equation:

b² – 4ac

When the discriminant is positive, b² – 4ac > 0, meaning the value of b² is greater than 4ac. This tells us that the quadratic equation has two distinct real roots.

How does this work in practice?

Let’s take an example:

x² + 5x + 6 = 0

In this equation, a = 1, b = 5, and c = 6.

The discriminant is: 5² – 4 * 1 * 6 = 1

Since the discriminant is positive, we know this equation has two distinct real roots. We can use the quadratic formula to find these roots:

x = (-b ± √(b² – 4ac)) / 2a

Plugging in the values, we get:

x = (-5 ± √1) / 2 * 1

This gives us two solutions:

x = -2
x = -3

So, a positive discriminant means that your quadratic equation has two distinct real roots. This makes solving the equation much easier and helps us understand the nature of the parabola it represents.

Let’s break down the concept of the discriminant and how it relates to real and complex solutions.

The discriminant is a part of the quadratic formula, which helps us solve quadratic equations (equations of the form ax² + bx + c = 0). The discriminant is calculated as b² – 4ac.

Here’s the key takeaway:

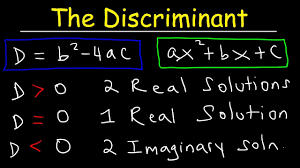
Positive Discriminant: A positive discriminant means you have two distinct real solutions. This is because you’re taking the square root of a positive number, which results in two possible values (one positive and one negative).

Zero Discriminant: A discriminant of zero means you have one real solution. This happens because the square root of zero is zero.

Negative Discriminant: This is where things get interesting! A negative discriminant means you’re taking the square root of a negative number. Since we can’t take the square root of a negative number within the realm of real numbers, we introduce complex numbers. Complex numbers involve the imaginary unit i, where i² = -1. Therefore, a negative discriminant leads to two complex solutions.

Think of it this way:

Imagine you have a quadratic equation that represents the path of a ball thrown in the air. The real solutions would be the points where the ball intersects the ground. If the discriminant is negative, the ball never touches the ground, meaning it stays in the air the entire time. This is where complex solutions come in; they represent points beyond the realm of “real” space.

Let’s use an example to solidify this:

Consider the equation: x² + 2x + 2 = 0.

Calculate the discriminant: b² – 4ac = (2)² – 4(1)(2) = -4
Interpret the result: The discriminant is negative, which means there are two complex solutions.

In summary, while a negative discriminant doesn’t produce real solutions, it does lead to two complex solutions. Complex numbers allow us to extend the concept of solutions beyond the real number system, offering a more complete understanding of quadratic equations.

Discriminant review (article) | Khan Academy

If the discriminant is negative, then the square root is not a real number. Square roots of negative values require the using of complex numbers. So, there are 2 solutions, that are not real numbers. Khan Academy

What does it mean if the discriminant of a discriminant is

In general, if the discriminant of a function $f(x)$ is a function $g(m)$, if the discriminant of $g(m)$ is always negative, then $f(x)$’s discriminant ($g(m)$) is Mathematics Stack Exchange

A Complete Guide to the Discriminant of Quadratic

A Negative Discriminant. A negative discriminant means that the value of b 2 – 4ac is less than zero. When using the quadratic formula the square root of a negative cannot be Maths at Home

If the discriminant of a quadratic equation is negative … – Cuemath

If the discriminant of an equation is negative, which of the following is true of the equation. Solution: A quadratic equation is an algebraic expression of the second degree in x. Cuemath

The Quadratic Formula: Solutions and the Discriminant

This relationship is always true: If you get a negative value inside the square root, then there will be no real number solution, and therefore no x-intercepts. In other words, if the Purplemath

Discriminant for types of solutions for a quadratic

Use the discriminant to state the number and type of solutions for the equation negative 3x squared plus 5x minus 4 is equal to 0. And so just as a reminder, you’re probably wondering what is the discriminant. Khan Academy

Quadratic Discriminant | Brilliant Math & Science Wiki

If \ ( \Delta < 0 , \) then the expression inside the square root is negative and the roots are both non-real complex roots. Also note that for a quadratic polynomial \ (ax^2 + bx + c ,\) Brilliant

The Discriminant | Intermediate Algebra – Lumen Learning

If the discriminant is positive, there are 2 2 real solutions. If it is 0 0, there is 1 1 real repeated solution. If the discriminant is negative, there are 2 2 complex solutions (but Lumen Learning