Let’s discuss the question: how to find the principal angle. We summarize all relevant answers in section Q&A of website Linksofstrathaven.com in category: Blog Finance. See more related questions in the comments below.
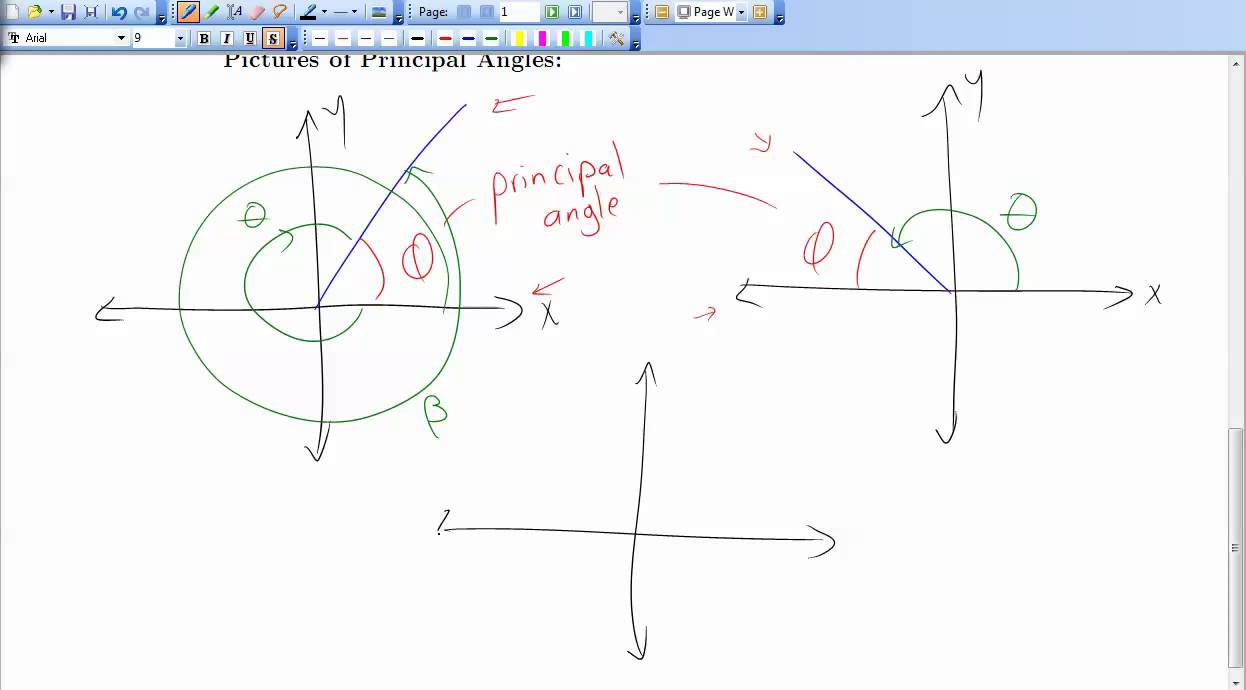
What is the principal angle in math?
Principal Angle – is the angle between oº and 360° (always a positive angle) Related Acute Angle – is an angle formed by the terminal arm of angle e in standard position. and the x-axis. – is ALWAYS positive and between 0 and 90°.
How many principal angles are there?
The principal angle is the first positive angle less than 360°. The terminal arm of an angle defines an infinite number of coterminal angles. These can be positive or negative and are defined in terms of the principal angle. They are multiples of 360°; that is, 360˚n, where n I.
Principal Angles
Images related to the topicPrincipal Angles

How do you write cosecant?
Cosecant is one of the main six trigonometric functions and is abbreviated as csc x or cosec x, where x is the angle. In a right-angled triangle, cosecant is equal to the ratio of the hypotenuse and perpendicular. Since it is the reciprocal of sine, we write it as csc x = 1 / sin x.
What is the reference angle of 540?
Subtract 360° 360 ° from 540° 540 ° . The resulting angle of 180° 180 ° is positive, less than 360° 360 ° , and coterminal with 540° 540 ° .
What are the principles of a angle?
In planar geometry, an angle is the figure formed by two rays, called the sides of the angle, sharing a common endpoint. Angles formed by two rays lie in a plane, but this plane does not have to be a Euclidean plane. Angles are also formed by the intersection of two planes in Euclidean and other spaces.
What is the cast rule?
The CAST Rule.
The CAST Rule says that in quadrant I all three of sinθ, cosθ, tanθ are positive. In quadrant II, only sinθ is positive, while cosθ, tanθ are negative. In quadrant III, only tanθ is positive, while sinθ, cosθ are negative.
Principal angles vs. Reference angles
Images related to the topicPrincipal angles vs. Reference angles

How do you write secant?
Secant (sec) – Trigonometry function
(See also Secant of a circle). In a right triangle, the secant of an angle is the length of the hypotenuse divided by the length of the adjacent side. In a formula, it is abbreviated to just ‘sec’.
What is Sinx times COSX?
Answer : The expression for sin x + cos x in terms of sine is sin x + sin (π / 2 – x). Let us see the detailed solution now.
Is sec 1 a sin?
tan x = sin x cos x . The cotangent of x is defined to be the cosine of x divided by the sine of x: cot x = cos x sin x . The secant of x is 1 divided by the cosine of x: sec x = 1 cos x , and the cosecant of x is defined to be 1 divided by the sine of x: csc x = 1 sin x .
What is the reference angle of 300?
360 – 300 = 60 degrees. The reference angle for 300 is 60 degrees.
Find Principal Angle for Trigonometric Ratio
Images related to the topicFind Principal Angle for Trigonometric Ratio
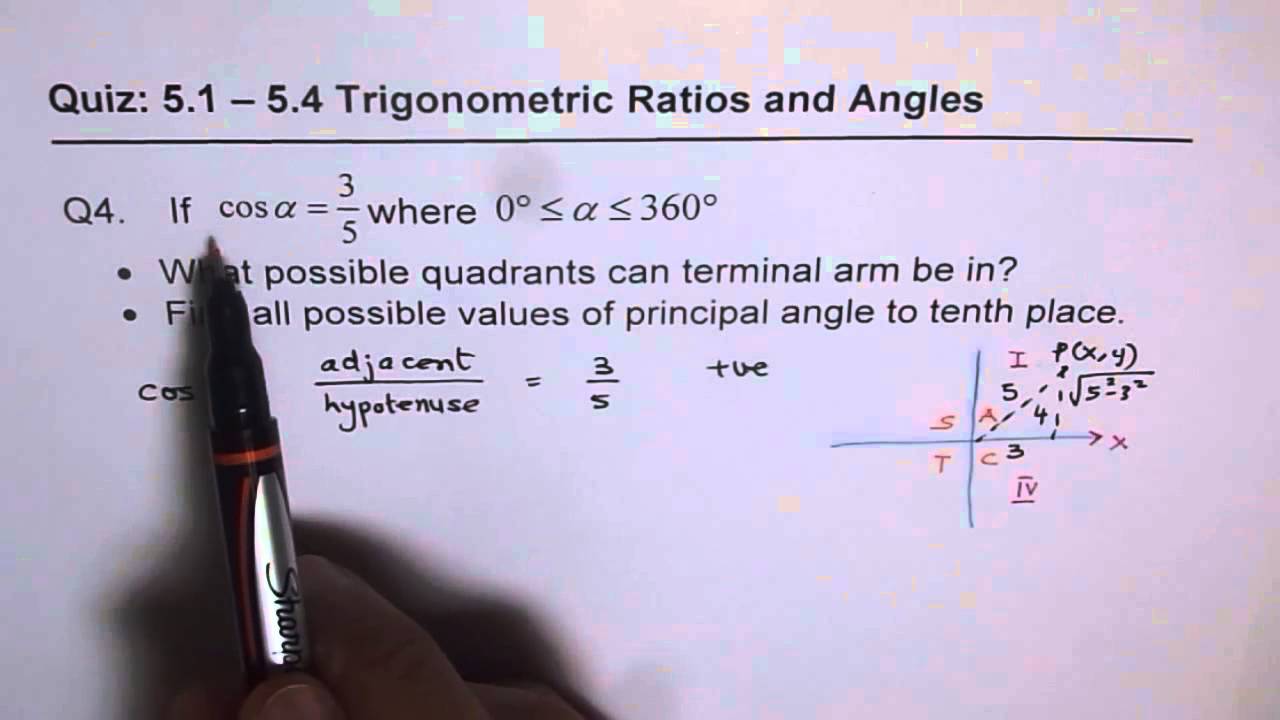
What is the reference angle of 120?
Reference angle for 120°: 60° (π / 3)
What is a 180 angle?
Angles that are 180 degrees (θ = 180°) are known as straight angles. • Angles between 180 and 360 degrees (180°< θ < 360°) are called reflex angles. • Angles that are 360 degrees (θ = 360°) are full turn.
Related searches
- how to determine the principal angle
- principal angle mohrs circle
- how to find the principal axis
- how to find the included angle
- principal angle and reference angle
- principal angle calculator
- principal angle of 540 degrees
- principal angle mohr’s circle
- how to find the equation of the principal axis
- how to find angle of principal stresses
- how to calculate principal angle
- how to find principal axis
- how to find reference angle
- principal angle of 9 radians
- principal angle and related acute angle
- principal angle between subspaces
Information related to the topic how to find the principal angle
Here are the search results of the thread how to find the principal angle from Bing. You can read more if you want.
You have just come across an article on the topic how to find the principal angle. If you found this article useful, please share it. Thank you very much.
